Author: J

Build New Functions From Existing Functions

J CCSS-Math Learning Progressions, HS Functions

This learning progression will be applied in a high school end of course class, and there will be no textbooks being used however there will some outside material included such as a Khan Academy video to supplement instruction. The common core state standards being aligned with this progression are: HSF.BF.B.3, HSF.BF.B.4, HSF.BF.B.4A, HSF.BF.B.4B, and HSF.BF.B.5. The following standards for mathematical practice are also included in the progression: MP1: Make sense of problems and persevere in solving them, MP2: Reason abstractly and quantitatively, and MP4: Model with mathematics.

In the first lesson, students investigate what effects adding a constant to a function might have, such as f(x) + k, k*f(x) or f(x+k). Students get more familiar with transforming, or building off of given functions. The second lesson will focus the students on trying to work in the opposite direction, finding the inverses of functions, and for our common core state standard we are sticking to simple linear functions. Students will learn how to prove algebraically if two functions are inverses of one another by taking the compositions of the functions. In the final lesson, students will take their new knowledge of inverses and investigate further the inverse property between exponents and logarithms.

Inverse

There will be a formative assessment at the end of the progression in the form of a short standards-based quiz that covers the cluster of standards. There are also worksheets for each lesson as well that are intended as benchmark assessments throughout the progression.

Learning Progression_TPA

Fun With Probability – 7th Grade

J CCSS-Math Learning Progressions, Common Core State Standards Mathematics, ML Statistics & Probability

This learning progression will be applied in a 7th grade mathematics classroom. The textbook being used throughout it will be; Core Connections – Course 2, by Dietiker, Kysh, Sallee & Hoey, specifically lessons 5.2.3-5.2.5. The common core state standards aligning with the lessons in the progression are:

  • CCSS.MATH.CONTENT.7.SP.C.8.A
    Understand that, just as with simple events, the probability of a compound event is the fraction of outcomes in the sample space for which the compound event occurs.
  • CCSS.MATH.CONTENT.7.SP.C.8.B
    Represent sample spaces for compound events using methods such as organized lists, tables and tree diagrams. For an event described in everyday language (e.g., “rolling double sixes”), identify the outcomes in the sample space which compose the event.
  • CCSS.MATH.CONTENT.7.SP.C.8.C
    Design and use a simulation to generate frequencies for compound events.

The following standards for mathematical practice are also included in the progression: MP1: Make sense of problems and persevere in solving them, MP4: Model with mathematics, MP5: Use appropriate tools strategically.

Multiple formative assessment techniques will be implemented in the progression to help students be more aware of their understanding and comprehension in relation to each of the standards and learning targets. Students are going to use learning logs throughout the learning progression and record personal entries summarizing their own understanding of the learning targets relative to each lesson. Also, exit slips/hinge questions will be used at the conclusion of each of the first two lessons of the progression as pre-cursors towards the next.

A sample instructional task that will be taking place in this progression is constructing probability tables through the use of an interactive game that students are sure to love! Attached is the complete learning progression including a narrative and complete activities/assessment tasks.

Learning_Progression_7th

GPE.B.4- Square; IM Assessment

J Assessment of CCSS-Math, Common Core State Standards Mathematics, HS Geometry

Alignment to Content Standards:

CCSS.MATH.CONTENT.HSG.GPE.B.4

Use coordinates to prove simple geometric theorems algebraically. For example, prove or disprove that a figure defined by four given points in the coordinate plane is a rectangle; prove or disprove that the point (1, √3) lies on the circle centered at the origin and containing the point (0, 2).

CCSS.MATH.CONTENT.HSG.GPE.B.5

Prove the slope criteria for parallel and perpendicular lines and use them to solve geometric problems (e.g., find the equation of a line parallel or perpendicular to a given line that passes through a given point).

Tasks

Plot these points on an x/y coordinate plane: A(4, 0), B(0, 3), C(-3, -1), and D(1, -4)

Coordinate Plane

1) Find the length of segment AB.

 

2) Find the slope of segments AB and CB.

 

3) Join the sides of quadrilateral ABCD. Prove that quadrilateral ABCD is a square. How do the answers in question 2 aid in this proof?

 

For the full commentary and solution to the task, open the following attachment:

Assessment Item

And the runner’s OUT! – CCSS.MATH.CONTENT.8.G.B.7

J Picture Problems

With the World Series recently wrapping up, what better way to get students engaged in math than through real world application with our nation’s pastime, baseball. If I were teaching a unit on geometry, I would engage students and create discussion by simply bringing up the current relevance of baseball. Salvador Perez was just named the MVP of the World Series and was the catcher for the winning Kansas City Royals (Perez is one example, a teacher could exemplify any catcher, maybe even the catcher on your professional team if you have student supporters.) Catchers are always on alert when there’s a base runner on first as they might try to steal second. A teacher can use these pictures to show some of the dimensions and geometric make-up of the field, also good for any students unfamiliar with the sport. They could then go on and prompt the question, “While the runner frDiamondom first only has to travel under 90 feet to reach second base with his lead off, how far does the catcher have to throw the ball to get the runner out at second?” Students could use the Pythagorean Theorem as is the state standard, or some may even be creative to see the isosceles triangle within the baseball infield’s dimensions and use one of the side lengths to determine the answer. Applying this back to the real world it can show how much of an advantage a runner may have or just how great a catcher has to be to consistently get runners out, such as Salvador Perez.

If student’s seemed interPerezested and they truly were engaged by the mathematical dynamics that makes up the game of baseball, teachers could build upon the dimensions of the field to prompt students towards higher level mathematics applications, for example using a runner’s actual speed and distance needed to travel to figure out how fast the baseball would have to be thrown to successfully throw the runner out (with a direct throw.) Teachers might also use the triangles found in the infield and speak upon congruency theorems!

CCSS.MATH.CONTENT.HSF.LE.A.2: Motion Detect Your Way to Exponential Discovery

J CCSS-Math Learning Progressions, HS Functions, Technology Teaching Issues

For students to truly connect with new ideas they need to be mentally engaged and curious in order for them to retain any knowledge. One way teachers can achieve this is having the students create their own problems and solutions as opposed to always just giving students problems out of textbooks, really having the students physically being a part of their learning through collecting data in person. This personal connection to the data will help them to achieve learning goals because they feel like they were a true part of the discovery, which they when actively performing their own experiments. Making use of technology is always a great way to get students excited, and in this activity in which students will discover exponential relations, a motion detector is used. I mean who would rather learn out of a textbook as opposed to using motion detectors to discover mathematics for themselves?

Students will be using Vernier CBR 2 motion detectors that hook up directly through CBRthe graphing calculators linking port, or for the TI-84 Plus, TI-84 Plus Silver Edition, TI-Nspire or TI-Nspire CAS, the CBR 2 can hook up through the USB port. When hooked up with a calculator it should automatically bring up the reading screen displaying the CBR and any distance detected by the sensor, with the options of: file, setup, start, graph, and quit. The technology is very intuitive making it fairly simple to use.

In this activity, students can be put into groups of about 5 to 6 and they will be testing the exponential pattern of rebound heights. They will be doing this using a small ball (bouncy ball, basketball, tennis ball, etc.) paired with the motion detector to measure the continuous height as the ball bounces. On a side note, have the students compete in their groups to see who can bounce the ball the best straight up and down, this should help deplete any problems of having balls flying all over the classroom and help to spark engagement of all students just as competition always seems to do best. This will also help to result in the most consistent and valid data, which is important in the activity. Group work also promotes cooperative skills needed for students to be successful and responsible citizens in a diverse society as they learn to work together, and to accept and critique ideas in order to come to a joint resolution.

Students will simply position the CBR 2 about a meter and a half over the ground and when the individual with the steadiest bounce is crowned in each group, they can position their ball at a starting point of a meter off the ground directly under the CBR 2 detector, then press start on the calculator screen and drop the ball. The graphing calculator will then graph the position of the ball. The first objective for th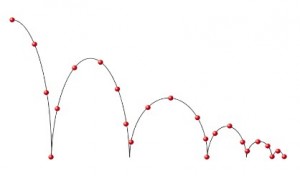Bouncee students is to derive the rebounding heights of the ball. When the graph on the calculator shows the time/distance relation, the students will see a parabolic trend pattern getting smaller and smaller, and they can use the “find maximum” tool under trace on their calculator to find the maximum height of the ball on each bounce.

Students will then record these heights in a table according to the number of bounces occurred, starting at 0 with the initial height determined (have all groups start with initial height at a meter), and so on until the ball was ultimately resting, about 5 or 6 bounces in. With the data entered into a table, both physically and into the table setting in the calculator, students can plot them onto a graph and discover the exponential pattern of the rebounding heights! The exponential function from the activity will be shown as y = Apx where y is the rebound height, A is the initial height (1 m), and x the number of bounces. This section of the analysis directly correlates to the common core standard: CCSS.MATH.CONTENT.HSF.LE.A.2
Construct linear and exponential functions, including arithmetic and geometric sequences, given a graph, a description of a relationship, or two input-output pairs (include reading these from a table). Students will also derive the value of p by plugging in the data derived in the experiment, which also directly correlates to the common core standard: CCSS.MATH.CONTENT.HSF.LE.B.5
Interpret the parameters in a linear or exponential function in terms of a context.

Students should be able to be able to more easily interpret the parameters of exponential functions and situations when being the ones who create them; positioning the ball at the initial height, and seeing how the function compounds (by number of bounces). When just giving students an equation such as y = Apx, most if not all would have any idea what any variable stood for. After performing this activity, students should be more successful in being able to determine the components and parameters in an exponential function and where each value comes from.

You can purchase the CBR 2 motion detector for the classroom on the Vernier website for $99 (http://www.vernier.com/products/sensors/motion-detectors/)

Decaying Bounce Worksheet

CCSS.MATH.CONTENT.8.F.B.5: 3-Act Math, Joulies

J HS Functions, HS Modeling, Technology Teaching Issues

Who hasn’t been enjoying a hot beverage just to burn your tongue while easy_link_Ltaking a drink? Most, if not all students, should be able to relate to this from previous real life experience. I found this “Joulies” activity from a list of Dan Meyer’s 3-Act math projects. In this project, students will be analyzing the relationship between the cooling temperatures of hot beverages, with and without the advertised product “Joulies”. Students will do so by using temperature probes to record both of the cooling temperatures graphically. Joulies are advertised as being able to cool a hot beverage such as coffee or tea to a perfect drinking temperature three times faster while also staying warmer twice as long, through the activity students will be able to determine the validity of this advertisement.

The temperature probes are a Vernier product (http://www.vernier.com) and Product image for Go!Tempwith the Vernier Easy Data App & Easy Link USB Interface it makes it very easy to use TI-84 plus calculators to hook our temperature probes up and record desired data. In the lesson plan and activity attached, you will read how this technology is involved in helping students achieve the common core state standard:

CCSS.MATH.CONTENT.8.F.B.5
Describe qualitatively the functional relationship between two quantities by analyzing a graph (e.g., where the function is increasing or decreasing, linear or nonlinear). Sketch a graph that exhibits the qualitative features of a function that has been described verbally.

 

Joulies Lesson Plan

Joulies Graphs